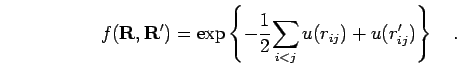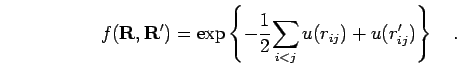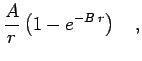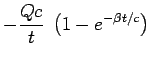Finite Temperature Jastrow Factor

The VDM method can be improved by including correlations that are missed in an Hartree-Fock type ansatz. This is usually done in form of a Jastrow factor f(R,R') as shown in Eq. 3.79,

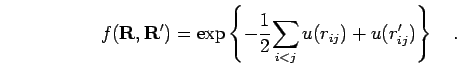$$f({\bf R},{\bf R}') = \exp \left \{ -\frac{1}{2}{\sum_{i<j}u(r_{ij})+u(r'_{ij})} \right\} \quad.$$ (263)

The Jastrow factor can be calculated at zero temperature using the RPA (see Ceperley and Alder (1981)), then generalized to finite temperature and approximately expressed in the form (Pollock, 2000),

$$u(r) = \frac{A}{r} \left( 1- e^{-B\,r} \right) \quad,$$ (264)

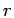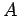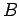where $r$ is the separation of the pair of particles. The coefficients $A$ and $B$ depend on density 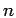$n$, inverse temperature 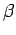$\beta$, and on the type of interacting particles. They are derive that the fulfill the cusp condition at any temperature. The coefficients for a pair of electrons is given by,

$$A_{ee} = c t \quad\quad,\quad\quad c=2 \sqrt{r_s^3/3} \quad\quad,\quad\quad t=\tanh \left( \beta/c \right)\quad,$$ (265)

$$B_{ee} = \sqrt{ 2/ A_{ee}} \quad.$$ (266)

For an electron and an ion of charge 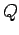$Q$, they read,

$$A_{ei} = - \frac{Qc}{t} \; \left( 1-e^{-\beta t/c} \right)$$ (267)

$$B_{ei} = \sqrt{ -4Q / A_{ei}} \quad.$$ (268)