Distribution of Permutation Cycles

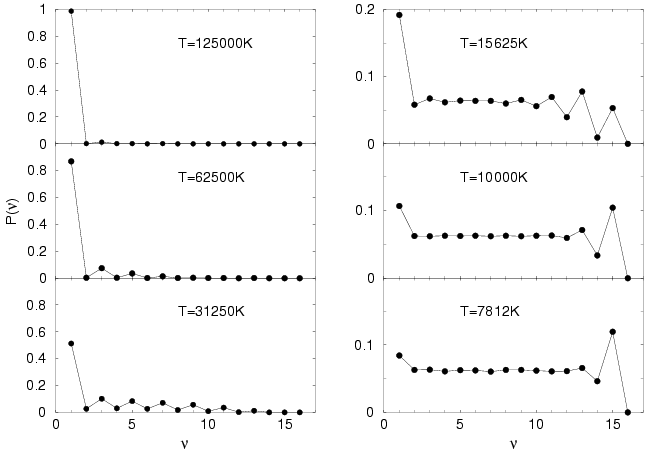
The distribution of permutation cycles has significant effects on the thermodynamics properties of the studied system since they represent the fermionic character. Here, we will discuss how it changes with increasing degeneracy.

Figure 2.4: Comparison of the cycle length distributions (probability of an electron being involved in a permutation cycle of the length $\nu$) in a PIMC simulation of hydrogen with $32$ protons and $16$ electrons of each spin state at $r_s=1.86$ for different temperatures $T$ using free particle nodes. Decreasing $T$ leads to an increased degeneracy and more uniform cycle distribution.

In Fig. 2.4, the probability distribution $P_\nu$ of permutation cycles of different length $\nu$ from PIMC simulations are shown. The normalization is given $\sum_\nu P_\nu=1$. We found that the fraction of $1$-cycles $P_1$ is a good candidate to discuss the degree degeneracy. Beginning at $1$ in a non-degenerate system, it decreases with increasing degeneracy. Simultaneously, states with higher cycle lengths are populated. At first, odd cycles have a higher probability, while one finds an almost uniform distribution at high degeneracy. The reason is that the nodal surfaces prohibit even permutations, which means an even number of even cycles must occur simultaneously and more importantly within the distance of the order of the thermal de Broglie wave length. It can be shown from the determinant that isolated even cycles would violate the nodes. This is also the reason why systems with even numbers of particles cannot form one long chain ($P_{16}=0$ in Fig. 2.4). At high degeneracy where the thermal de Broglie wave length is larger than the inter-particle spacing, the nodal constraint does not discriminate between even and odd cycles.

This observed cycle distribution with fermion nodes is very different from what one expects from direct fermion methods, where one considers the signs explicitly and does not use nodal surfaces, e.g. the cycle distribution of a system of non-interacting fermions can be calculated in the grand canonical ensemble (Feynman, 1972). One has to differentiate between odd and even cycle lengths leading to positive and negative contributions to the partition function. Incorporating the sign into $P_\nu$, it reads

$$P_\nu = (-1)^\nu \; \frac{1}{n} \; \frac{e^{\nu \mu \beta}}{(4 \pi \lambda \beta \nu)^{3/2}}\;,$$ (114)

where the chemical potential $\mu$ for given $\beta$ and density $n$ is determined by the normalization $\sum_{\nu=1}^\infty P_\nu=1$. $P_\nu$ is the rapidly decaying function of $\nu$, which has little in common with observed cycle distributions from restricted path integrals.